Only distances are required to reconstruct submanifolds
Résumé
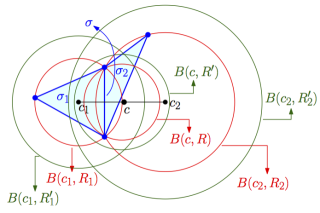
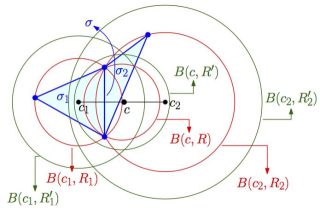
In this paper, we give the first algorithm that outputs a faithful reconstruction of a subman-ifold of Euclidean space without maintaining or even constructing complicated data structures such as Voronoi diagrams or Delaunay complexes. Our algorithm uses the witness complex and relies on the stability of power protection, a notion introduced in this paper. The complexity of the algorithm depends exponentially on the intrinsic dimension of the manifold, rather than the dimension of ambient space, and linearly on the dimension of the ambient space. Another interesting feature of this work is that no explicit coordinates of the points in the point sample is needed. The algorithm only needs the distance matrix as input, i.e., only distance between points in the point sample as input.
Fichier principal
 only-distances.pdf (895.98 Ko)
Télécharger le fichier
only-distances.pdf (895.98 Ko)
Télécharger le fichier
 only-distances-fig.png (94.31 Ko)
Télécharger le fichier
only-distances-fig.png (94.31 Ko)
Télécharger le fichier
 only-distances-fig.jpg (41.14 Ko)
Télécharger le fichier
only-distances-fig.jpg (41.14 Ko)
Télécharger le fichier
Origine : Fichiers produits par l'(les) auteur(s)
Format : Figure, Image
Origine : Fichiers produits par l'(les) auteur(s)
Origine : Fichiers produits par l'(les) auteur(s)
Format : Figure, Image
Origine : Fichiers produits par l'(les) auteur(s)
Origine : Fichiers produits par l'(les) auteur(s)
Loading...